Cumbersome Division
In my earlier post “Accuracy vs Precision” I mentioned an issue that crops up from time to time. What do you do when you have to equally divide a line with an awkward (39-5/16”) or unknown length into a difficult number of parts (7)? No problem in this modren age, right? Just whip out the old Construction Master™ tap a few keys and bingo there's your number 5-5/8”. In order not to have to calculate (in your head_ 10-10/8” = 11-2/8” = 10-1/4”. . . 15-15/8” = 17-7/8. . . . . . you set a compass/divider to exactly 5-5/8” and set about stepping off your seven divisions. Except when you get to the last one you discover you're almost 1/8” out. WTF?

Don't get me wrong. I LOVE my Construction Master™. Could not live without it. But, it is lying to you (for your own good.) In actuality, seven EQUAL divisions of 39-5/16” is precisely 5-81/132”. In order not to make you crazy with a number you can't measure; it rounds to the nearest 1/16”. The more divisions you have, the worse the problem gets; because the rounding error accumulates with each repetition.

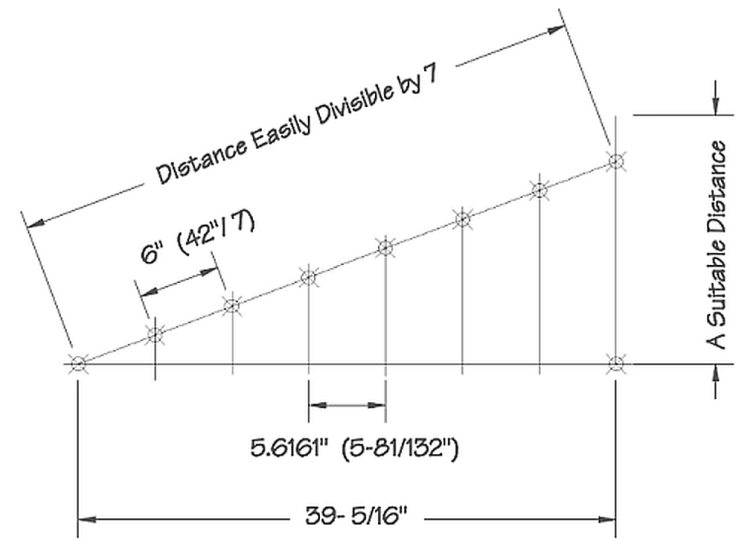
There is a gag to beat this problem. You can use it to precisely mark out any number of equal divisions of your odd length line. You can also mark out unequal divisions as long as they're all multiples of the smallest one. Let me step you through it using the example right.

At one end of the line erect a perpendicular of a suitable length.
From the other end look for a diagonal that is easily divisible by the number of divisions you want; as it passes through the perpendicular.
Tick off the the chosen increments along the diagonal.
Perpendiculars, from the base line, which pass through these ticks gives you a precise division of the line into seven exactly equal parts.

I had thought I'd gotten this gag out of the Backtage Handbook; but The Backstage Handbook shows a different construction method for the equal division of a line; involving multiple parallel line constructions.